Talking Math: How to Engage Students in Mathematical Discourse

The importance of engaging students in meaningful mathematical discussion has long been identified as an essential component of students’ mathematics learning.

When students share and exchange their ideas, both they and their teachers benefit.

Dr. Gladis Kersaint

Understanding the Importance of Mathematical Discourse

Talking about mathematical concepts allows students to reflect on their own understanding while making sense of and critiquing the ideas of others. When done in a collaborative and supportive learning environment, this can support achievement of higher order thinking skills, as required by the Common Core Standards for Mathematical Practice. Students can make conjectures, link prior knowledge to current understanding, reason about mathematics, refine and amend their approaches, and take ownership of their mathematical knowledge.

Students benefit greatly from learning to use the tools of mathematical discourse—including words, symbols, diagrams, physical models, and technology—to present and defend their ideas. Imparting these new skills on students allows teachers to access, monitor, and evaluate students’ mathematical understanding and development.

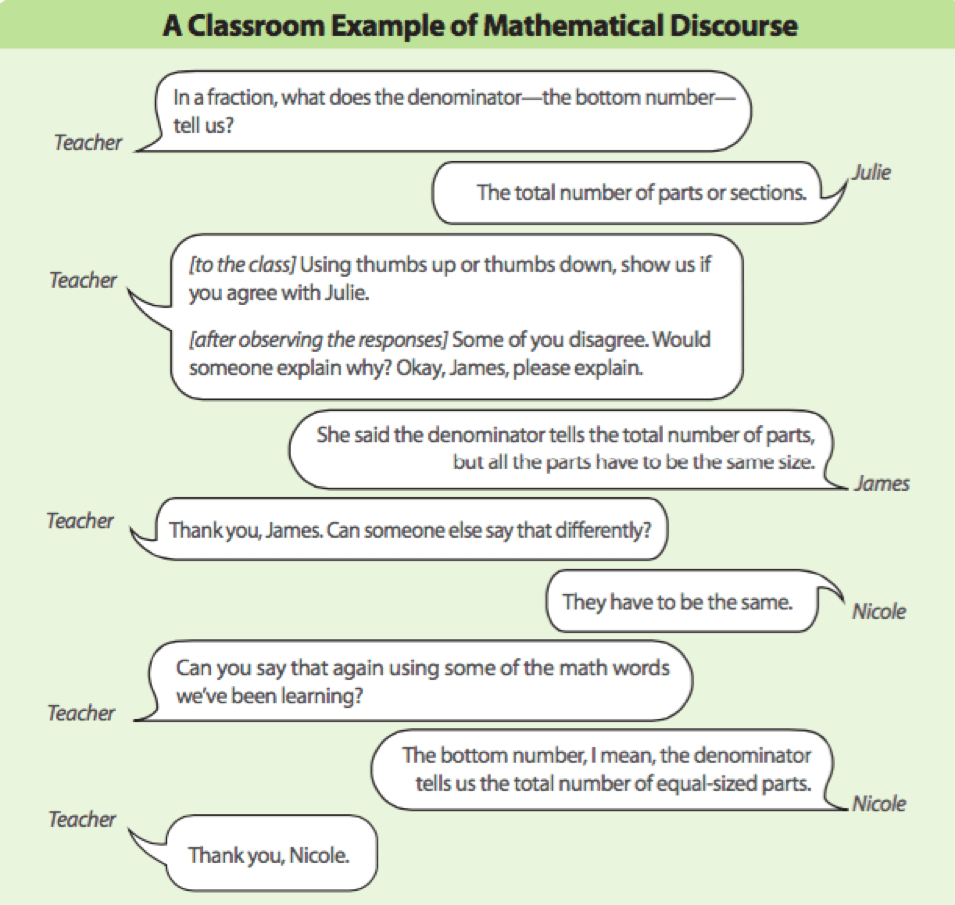
Mathematical Discourse in Action

One approach to giving students the information needed for a mathematics lesson involves the teacher simply telling the students what they need to know. Far more productive, however, is more a thoughtful approach to student engagement that encourage a productive dialogue, as seen in the example below. As educators, we need to structure lessons to encourage student interaction, address gaps in student understanding, and help students express mathematical concepts more precisely. Providing opportunity for conversation does just that.

Does this look like a classroom dialogue you’ve seen before? To further your understanding of the importance of facilitating mathematical discourse, ask yourself:

• How did these students benefit by sharing what they knew as part of the mathematics discussion?
• Why did the teacher allow students to evaluate the correctness of Julie’s answer?
• Why did the teacher ask another student to restate what James said?

Establishing a Discourse-Rich Mathematics Learning Community

To engage students in productive mathematics discussions, it is important to establish a learning environment that welcomes student involvement. The first step is setting the expectation that every student will contribute to the discourse community.

Students must be encouraged to use their problem-solving, reasoning,and communication skills to make conjectures, explore their own ideas and approaches, and find solutions to routine and non-routine mathematics problems.

In particular, the five process standards–problem solving, reasoning and proof, communication, connections, and representation–can be seen in action in a discourse-rich mathematics community as students interact, question one another and convey their understanding. During this dialogue, teachers can recognize errors in context and reinforce that they are natural occurrences that enhance learning.

What does a classroom rich with mathematical discourse look like? Walk into one and you’ll likely see:

• Students and teachers acknowledging and discussing errors and the reasons behind them to help students build greater understanding.
• Students questioning each other using mathematics arguments to establish the correctness of solutions.
• Students reaching and justifying conclusions based on their own mathematics knowledge without relying on the authority of teachers.
• Students engaging in “productive struggle” with appropriate scaffolds for support.
• Students encouraged to use a variety of approaches to convey their knowledge and solution strategies, including oral presentations; written explanations; and physical, graphical, pictorial, or symbolic representations.

Understanding Student and Teacher Roles in Mathematical Discourse

Facilitating student engagement in mathematical discourse begins with the decisions teachers make when they plan classroom instruction. The tasks they use, the ways in which they organize the classroom, and the behaviors they model communicate expectations for classroom norms, including the ways students are expected to engage in classroom discussions.

Depending on prior experiences, students might find these new expectations for engagement uncomfortable and may not be ready to plunge into mathematical dialogue feet first. Teachers must ease the transition to a dialogue-rich mathematics classroom and prepare students to engage in such discussions. It is also important to carefully consider the best ways to coordinate student interaction in pairs, small groups, or whole-class interactions to ease transitions and maximize learning.

To support students, teachers must help students create avision for expected behaviors and actions, prepare them for their roles by modeling or role-playing, and reinforce these behaviors consistently. Teachers need to monitor progress as students engage in mathematics discussions, supporting them as their mathematical knowledge grows and they become more skilled at expressing ideas coherently and using vocabulary, syntax, and semantics precisely.

Teachers should observe, listen to and monitor students to support instructional decision-making. Determining what questions to ask, which students to call on, when to intervene and when to extend student thinking provides opportunities to understand student thinking, monitor growth and assess knowledge. A discourse-rich classroom enables teachers to gain insights not only about what students know, but also about the approaches they use, how–and how well–they understand the ideas, and the ways they present their knowledge.

In addition to content knowledge, mathematical discourse allows teachers to monitor students’ dispositions and gauge their developing confidence, interest, and perseverance. Teachers can use this information to determine areas of confusion or frustration in order to decide when an intervention might be needed. They also examine understandings and misconceptions revealed during classroom discussions and adjust lesson plans accordingly.

Asking the right questions can help support instructional decision making and direct student focus. Some examples are below:

Engaging Every Student in Mathematical Discourse

All students are mathematics language learners, regardless of their level of English language proficiency, and discourse allows ALL students to develop mathematical language.

While it is important to build scaffolds to support students who are learning English and mathematics at the same time, it is essential to recognize that mathematics is a technical language that all students must learn. Students who are English speakers also require support as they learn the language of mathematics.

As outlined in the Standards for Mathematical Practice, students should become fluent in mathematical language, including vocabulary, symbolic representations, syntax, semantics, and linguistic features. In addition, they must have ample opportunities to use the language of mathematics as they engage in various forms of communication. Discourse allows students to practice precision in multiple areas, including:

1.Vocabulary

• English words with a different meaning in mathematics, such as “negative,” “table,” or “rational.”
• Specialized terms, such as “hypotenuse” or “trapezoid.”
• Terms with multiple meanings in mathematics, such as “median” or “base.”

2. Symbols

• Ways to read and interpret symbolic representations. For example, “a × b” can be expressed as “a times b,” “the product of a and b,” or “multiply a and b.”

3. Syntax

• Understanding the rules that govern the structure of sentences. For example, “A number y is 4 more than a number x” is translated symbolically to “y=x+4.”

4. Semantics

• The process of making meaning from language.
• The same mathematics word may be interpreted differently depending on the context. For example, the median of a set of numbers versus the median of a triangle.

Teachers support mathematical language development by asking key questions and encouraging students to ask for clarity. By doing this, teachers motivate and encourage students and facilitate productive discussion, fostering a supportive classroom culture that maximizes learning.

This blog is part of a three post series on the importance of mathematical discourse from Curriculum Associates, a Getting Smart Advocacy Partner, and Dr. Gladis Kersaint, the author of the recently published whitepaper Orchestrating Mathematical Discourse to Enhance Student Learning. Download your free copy here. 

For more on Curriculum Associates, check out:

• Talking Math: 100 Questions That Help Promote Mathematical Discourse
• 6 Strategies For Getting Students to Engage in Mathematical Discourse
• Curriculum Associates: Leveraging For-profit Power With a Nonprofit Purpose

Dr. Gladis Kersaint is the Dean of the Neag School of Education at the University of Connecticut and an author of the Ready® Mathematics and Ready® Classroom Mathematics programs from Curriculum Associates.

Stay in-the-know with all things EdTech and innovations in learning by signing up to receive the weekly Smart Update.