Smart Balances in Smart Blends

By: Jason Zimba
It can be a difficult challenge for teachers to meet students where they are and also teach grade-level mathematics. Negotiating that tension is the subject of this post, in which I’ll offer some thoughts on striking smart balances in rotation models. I’ve also included thoughts from two mathematics teachers, Allyson (“Ryan”) Redd of North Carolina and Peter Tang of Tennessee.
One condition for striking a smart balance comes from the K-8 Publishers’ Criteria for the Common Core State Standards for Mathematics . There it is stressed that the mathematics curriculum should give all students extensive work with grade-level problems. This should be true for blended classrooms as well as traditional classrooms.
Why is this important? Instructional coaches are familiar with the phenomenon of “fractions forever.” Students who leave elementary school without a solid grasp of fractions can end up “getting the fraction treatment” from sixth grade onwards. The treatment continues year after year, despite its lack of success, and in the meantime the student has lost the opportunity to learn algebra.
As teachers know, unfinished learning from earlier grades is normal and prevalent. It shouldn’t be ignored, but it also shouldn’t be used as a basis for canceling grade-level work. Fortunately, middle-grades mathematics offers opportunities to handle unfinished learning in the context of the grade level. For example, instead of turning an eighth-grader around and frog-marching him back to fractions (or, worse, airbrushing fractions out of the algebra textbook), algebra problems could become occasions for some students to practice weak fraction skills and revisit fraction concepts in a new light (such as the idea that fractions are quotients, for example). Ideally, rotation models could provide individualized help in the context of grade-level content.
A related boundary condition is that rotation models should regularly allow time for the whole class to concentrate together on the same well-designed, carefully sequenced problem. This is a mode of classroom work in high-performing Japan. Inevitably, some students in the class will use clumsier methods to solve the problem–perhaps concrete approaches based on much earlier mathematics. Other students will use more abstract or powerful methods, closer to the new methods that the problem is aiming toward. The teacher then helps students in the class to understand each other’s methods. Thus, the teacher doesn’t see it as his job to give each student “just the right problem” for him or her at that point in time; all of the students are working on the same problem. Nor, however, does the teacher ignore the very real variability in his classroom. Indeed, the teacher sees it as his job to decrease the variability in students’ approaches, in such a way that every student moves toward the mathematical goals of the lesson. That is roughly what it means, in such a system, to say that you are teaching the class the mathematics of the grade.
Ryan Redd, an instructional coach and eighth-grade mathematics teacher, sees the value of this approach. She says, “Students benefit from seeing multiple ways to solve a problem. Sometimes with rotation models there is only one view of a problem, and the student might not be able to access the problem in that particular way.”
What happens when individual student needs become extreme? Peter Tang teaches seventh-grade 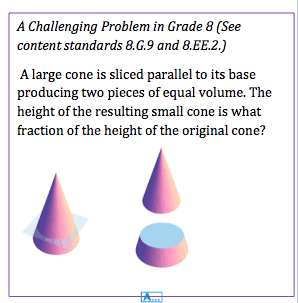mathematics in a school where a significant number of the students are behind by three or more grade levels. He says, “I work hard to scaffold grade-level work for these students, but it’s a struggle.” Peter uses a rotation model to handle the extreme differences in his classroom, stressing the benefits: “Essentially, rotation lowers my class size, which makes it easier to have conversations with my students about academic content.
Personalization has a role for the most able students as well. One thing high-performing students need is challenging problems–and that doesn’t necessarily mean out-of-grade-level content. Problems that are challenging for highly able students can be created based on grade 8 content (see illustration), and it would be helpful for rotation models to leverage this fact.
A final boundary condition: ensure that the large majority of students’ time is devoted to major work. New technologies and digital tools offer exciting opportunities for individualized and nonlinear paths, but they should not turn the curriculum into a random walk through the content landscape. The strong focus of the Common Core on arithmetic in grades K-5, and the coherent progressions to algebra in grades K-8, are the north star for bringing students to college- and career-readiness and STEM readiness in mathematics. No blend is smart enough to compensate for a mile-wide, inch-deep curriculum.
This blog is brought to you by The Nellie Mae Education Foundation as part of a series on blended math. For more stayed tuned for the Getting Smart on Blending Middle Grade Math bundle and see the other posts in this series:

• Reflections on a Khan Blend
• Lessons from Math KIPP Blends
• Middle School Math Chat: Connections are Key
• Summer School: A Great Time to Try Blended Learning in Middle School Math
• Make or Break, Why Middle School Math Counts

Jason Zimba was a lead writer of the Common Core State Standards for Mathematics and is a Founding Partner of Student Achievement Partners.